Quantum Zeno Effect – A Watched Pot Never Boils

Quantum physics is a jungle of paradoxes. Any direction you go you end up with a paradox, waiting to pounce on you, its claws ready to slash through your common sense, making you wonder what is actually real, and ridiculing at your inability to comprehend it. Most of these paradoxes are not really paradoxes but they just showcase our inability to perceive quantum physics. Be it the Schrodinger’s cat paradox or any other, ultimately they have an explanation. Generally, we oversee and ignore it, until one day when we finally realize that we are completely wrong in our perception of it.

Quantum Zeno effect is a very interesting consequence of the mysterious quantum world. Before diving deeper into the effect, have a look at the classical counterpart of the same.

The Zeno Paradox:

Zeno of Elea was a Greek philosopher. He gave paradoxes which went against one’s common sense and made one question about the reality of his perception. One of his famous paradoxes is the arrow paradox:

If everything, when it occupies an equal space, is at rest at that instant of time, and if that which is in locomotion is always occupying such a space at any moment, the flying arrow is therefore motionless at that instant of time and at the next instant of time but if both instants of time are taken as the same instant or continuous instant of time then it is in motion.
— as recounted by Aristotle, Physics VI:9, 239b5, Wikipedia.

Zeno states that for the motion to occur, the object must change its position which it occupies. For an arrow in flight, at a given instant of time, it can not be moving at all because time is not elapsing. Hence it must occupy the same position always. Thus if everything is motionless at a given instant, and if time is composed of many instants, then no motion must be possible.

In short, he states in his paradox that a flying arrow that is being observed can never fly.

This, even though it is counterintuitive, it is only a vague attempt to crack open our common sense. Ultimately it is just an argument and not a firm physical statement.

The Quantum Zeno Effect

This has inspired a beautiful effect in quantum physics, the quantum Zeno effect: An observed water pot in the quantum world never boils. Or to be put in a better way, a quantum system cannot undergo any changes while it is being observed.

This is not a paradoxical statement like Zeno paradox but is actually an observed and proven quantum effect. But to understand this paradox, you first need to know what is meant by observation in the quantum world, and how states evolve with time.

Quantum states and their evolution:

Consider a quantum system, which upon observed or measured gives one of the two possible values, either A or B. The state of the system is represented by |𝜓〉. Let us say that the system is in state |A〉if the measurement gives the value A and it is in state |B〉 if the measurement gives value B.

Quantum Superposition, which is the heart of quantum mechanics states that at any time, the system is in a superposition of all possible states. Mathematically it can be written as  |𝜓〉= C₁|A〉+ C₂|B〉, where C₁ and C₂ are two complex numbers, subject to the condition C₁² + C₂² = 1.

The system which is in the state |𝜓〉, when measured, wavefunction collapses. The collapse of wavefunction means that the system which is in superposition goes back to one of the pure states |A〉or |B〉. Thus, upon measurement, the system goes back to state |A〉 with probability |C₁²|, and to state |B〉with probability |C₂²|.

If you start with the state |𝜓〉=|A〉, the energy content of the system drives it towards superposition. So at a later time t, the system will be in state |𝜓〉=C₁(t) |A〉+ C₂(t) |B〉. This is how systems evolve in quantum mechanics.

The Zeno Effect

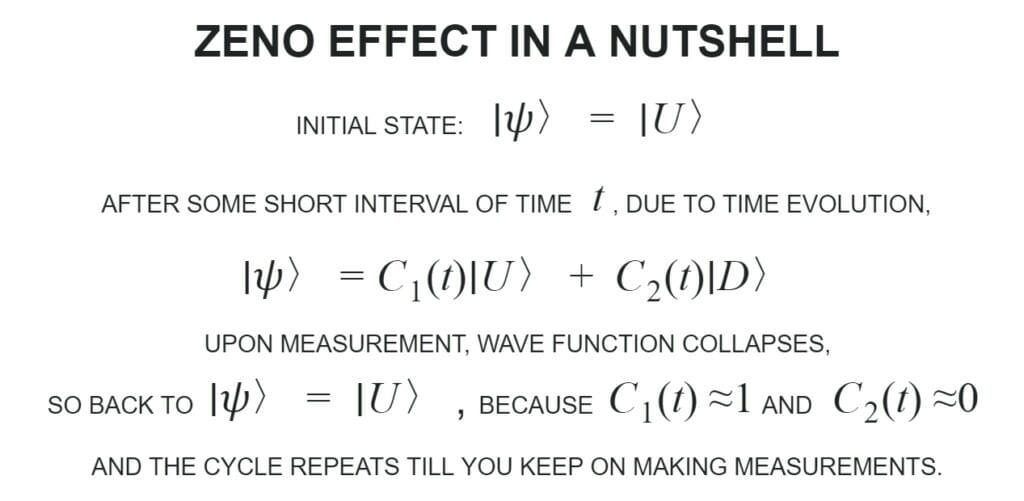
Now coming to our Zeno effect, let’s take a radioactive atom. This atom can be in either of the two possible states – Decayed or Undecayed. Let’s represent the decayed state by |D〉and the undecayed state by |U〉. Once we observe the system, we see either the atom is undecayed (in state |U〉) or it is undecayed (in state |D〉).

Initially, we take an undecayed atom. The atom is in state |𝜓〉= |U〉. Since the atom has some energy, it evolves continuously, ending up in the superposition state |𝜓〉=C₁(t) |U〉+ C₂(t) |D〉. Thus a measurement at a later time will give a decayed atom with probability |C₂(t)²| and undecayed atom with probability |C₁(t)²|.

Suppose initially the radioactive material was in an undecayed state, i.e C₁(t=0) =1and C₂(t=0) =0 . Now if we make a measurement on it after a very short interval of time t, then the system is most likely to be in the same undecayed state. This is because a very short period of time has elapsed and the system has not evolved much, meaning C₁(t) ≈1and  C₂(t)≈0.

So, each measurement you make will take the atom back to its undecayed state restarting the whole process. Which means that continuous measurement at small intervals of time will restrict any changes in a system. Even a radioactive atom having a mean life of 5 seconds, can be prevented from decaying for as long as we want, for minutes, hours, days or even years.

So indeed in the quantum world, observed water pot never boils. A system can never undergo any changes when it is being observed continuously at short intervals of time. Even though it seems to be totally weird and wrong, it is indeed a physical effect which has been proven experimentally. But this effect requires distinguishable quantum states, and this is not the case in the classical world, where all states are continuous. Thus this effect is not observable in the classical world.

Read other articles related to Science only at thehavok.com